|
Michelle E. Chung |
*
EMAT6680 Final Assignment A: Bouncing Barney |
|
Barney is in the triangular room shown here.
He walks from a point on BC parallel to AC.
When he reaches AB, he turns and walks parallel to BC.
When he reaches AC, he turns and walks parallel to AB.
Prove that Barney will eventually return to his starting point.
How many times will Barney reach a wall before returning to his starting point?
Explore and discuss for various starting points on line BC, including points exterior to segment BC.
Discuss and prove any mathematical conjectures you find in the situation.
|
 |
First, let's construct the room and think about Barney's trail.
|
| Room & Barney's Trail
Construction |
|
|
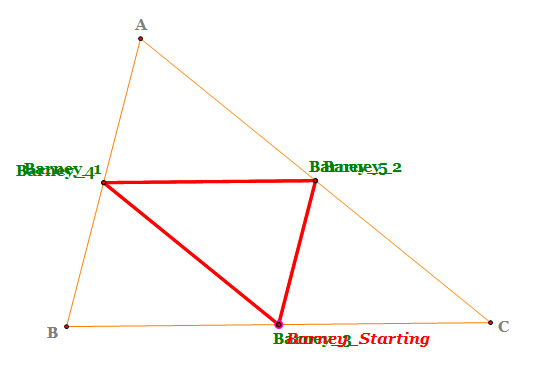
This is the picture of the room that Barney walks and his trail.
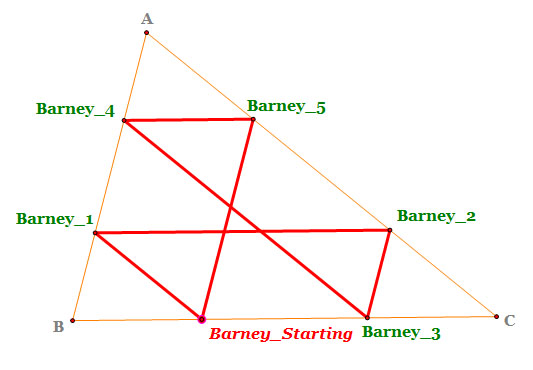
* If you want to see his trail in GSP movie, please click here. >>> Barney's Trail Movie
|
Reference
|
- Barney starts from point Barney_Start.
- Each line segment in the room, which is triangle ABC, is parallel to each corresponding side.
- His trail is 'Barney_Start -> Barney_1 -> Barney_2 -> Barney_3 -> Barney_4 -> Barney_5 -> Barney_Start'.
- As we see, he returns back to his starting point.
|
Back to the Top |
Now, let's prove that Barney will eventually return to his starting point.
|
|
Case 1
: when Barney_Start is not on the trisecting point of line segment BC, midpoint of line segment BC, nor one of the vertex (point B or C) |
|
|
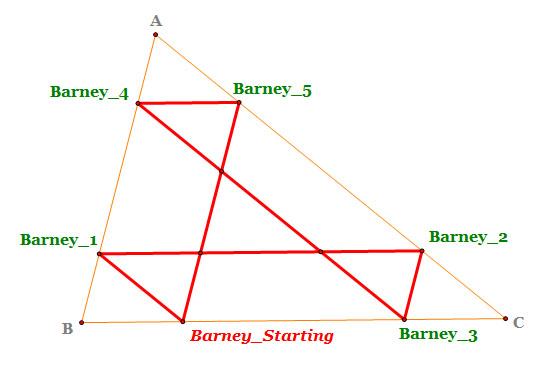
1. Consider triangle B_1 B_S E, triangle F B_3 B_2, and triangle B_4 D B_5.
- Since line segment B_4 B_3 is parallel to B_1 B_S, and line segment B_1 B_S is transversal,
m(angle B_2 F B_3) = m(angle E B_1 B_S) by the definition of Corresponding Angle.
Similarly, m(angle B_2 F B_3) = m(B_5 B_4 D) by the definition of Corresponding Angle.
- Since line segment B_5 B_S is parallel to B_2 B_3, and line segment B_4 B_3 is transversal,
m(angle B_4 D B_S) = m(angle F B_3 B_2) by the definition of Corresponding Angle.
Similarly, m(angle B_4 D B_5) = m(B_1 B_S E) by the definition of Corresponding Angle.
- So, triangle B_1 B_S E, triangle F B_3 B_2, and triangle B_4 D B_5 are similar.
2. Consider triangleC B_2 B_3 and triangle F B_3 B_2.
- Since line segment AC is parallel to line segment B_4 B_3, and line segment B_2 B_3 is transversal,
m(angle F B_3 B_2) = m(angle C B_2 B_3) by the definition of Alternate Interior Angle and
m(angle F B_2 B_3) = m(angle C B_3 B_2) by the definition of Alternate Interior Angle.
Also, line segment B_2 B_3 is the common side of two triangles.
So, triangleC B_2 B_3 and triangle F B_3 B_2 are congruent.
- So, line segment C B_2 and line segment F B_3 are congruent.
3. Consider quadrilateral B_S B_3 B_2 E.
- Since line segment E B_2 is parallel to line segment B_S B_3 and line segment E B_S is parallel to line segment B_2 B_3,
quadrilateral B_S B_3 B_2 E is a parallelogram.
- So, line segment B_S B_3 and line segment E B_S are congruent.
- Thus, triangle F B_S B_2 and triangle B_1 B_S E are congruent.
4. Consider triangle E B_1 B_S and triangle B B_2 B_1.
- Since line segment AB is parallel to line segment B_5 B_S, and line segment B_1 B_S is transversal,
m(angle E B_1 B_S) = m(angle B B_S B_1) by the definition of Alternate Interior Angle and
m(angle E B_S B_1) = m(angle B B_1 B_S) by the definition of Alternate Interior Angle.
Also, line segment B_1 B_S is the common side of two triangles.
So, triangleB B_S B_1 and triangle E B_1 B_S are congruent.
- So, m(angle B_1 B B_S) = m(angle B_S E B_1).
5. Suppose that Barney doesn't come back to the starting point, which is B_S.
- Then there is point B_F on line segment BC, which is the intersecting point of the last(?) trail from B_5 and line segment BC.
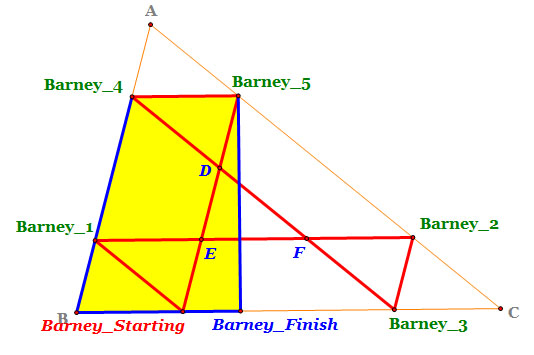
6. Consider quadrilateral B B_F B_5 B_4.
- Since line segment B_4 B_5 is parallel to line segment B B_F and line segment B_4 B is parallel to line segment B_5 B_F,
quadrilateral B B_F B_5 B_4 is a parallelogram.
- So, m(angle B_F B B_1)=m(angle B_4 B_5 B_F).
- Since m(angle B_1 B B_S) = m(angle B_S E B_1) and m(angle B_S E B_1) = m(angle D B_5 B_4),
m(angle B_4 B_5 B_F) = m(angle D B_5 B_4);
however, as we see, m(angle B_4 B_5 B_F) = m(angle B_4 B_5 B_S) + m(angle B_S B_5 B_F).
So, in order for m(angle B_4 B_5 B_F) = m(angle B_S B_5 B_4) to be true, m(angle B_S B_5 B_F) = 0.
- Hence, B_S = B_F.
This is contradiction.
- Therefore, Barney has to come back to the starting point, Barney_Start.
|
Case 2
: when Barney_Start is on the trisecting point of line segment BC
|
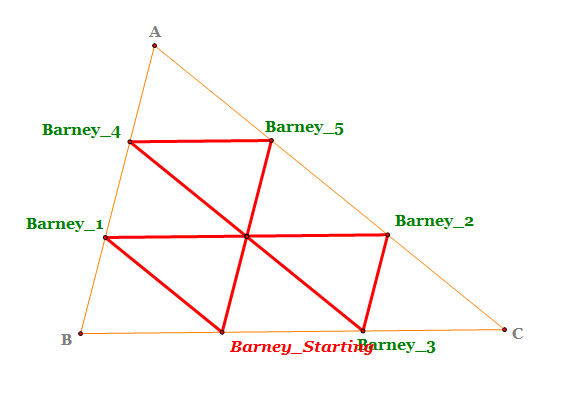
- This is almost same case as Case1, and we can use same proof.
|
Case 3
: when Barney_Start is on the midpoint of line segment BC |
|
1. Suppose that point B_S is the midpoint of line segment BC.
- Then point B_S = point B_3.
- Since point B_S is the midpoint of line segment BC and line segment B_1 B_S is parallel to line segment AC,
point B_1 = point B_4 and point B_1 is the midpoint of line segment AB.
- Similarly, since point B_1 is the midpoint of line segment AB and line segment B_1 B_2 is parallel to line segment BC,
point B_2 = point B_5 and point B_2 is the midpoint of line segment AC.
- So, triangle B_S B_1 B_2 is the medial triangle of triangle ABC.
2. Suppose that Barney doesn't come back to the starting point, which is B_S.
- Then there is point B_F on line segment BC, which is the intersecting point of the last(?) trail from B_2 and line segment BC.

- However, since pont B_2 is the midpoint of line segment AC and line segment B_5 B_F is parallel to line segment AB,
point B_F should be a midpoint of line segment BC.
- Hence, B_F = B_S.
This is contradiction.
- Therefore, Barney has to come back to the starting point, Barney_Start.
|
Case 4
: when Barney_Start is on the one of the vertex (point B or C) |
|
|
Back to the Top |
How about the number that Barney reach a wall before returning to his starting point?
|
Case 1 & 2 |
|
|
As we count, it is 6 times,
i.e. Barney will reach a wall 6 times before returning to his starting point, Barney_Start.
|
Case 3 |
As we count, it is 3 times,
i.e. Barney will reach a wall 3 times before returning to his starting point, Barney_Start.
|
| Case 4 |
|
Actually, in this case, it is hard to say the number of a wall that he reach because he is walking on the wall(maybe impossible in the real world...); however, if we assume that he reaches a wall when he reaches a vertex of the triangle, it would be 3 times.
|
Back to the Top |
Let's explore and discuss for various starting points on line BC, including points exterior to segment BC.
| Case 1 & 2
: when Barney_Start is interior to segment BC except at the midpoint
|
|
|
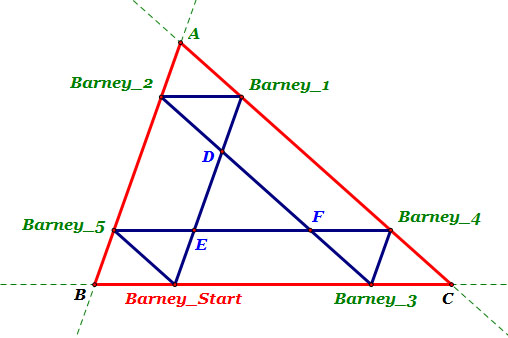 |
Conjecture
- The sum of length of Barney's trail is equal to the perimeter of triangle ABC.
|
|
Case 3
: when Barney_Start is at the midpoint of segment BC |
 |
Conjecture
- The sum of length of Barney's trail is equal to half of the perimeter of triangle ABC.
|
|
Case 4
: when Barney_Start is on the end point of segment BC |
 |
Conjecture
- The sum of length of Barney's trail is equal to the perimeter of triangle ABC.
|
|
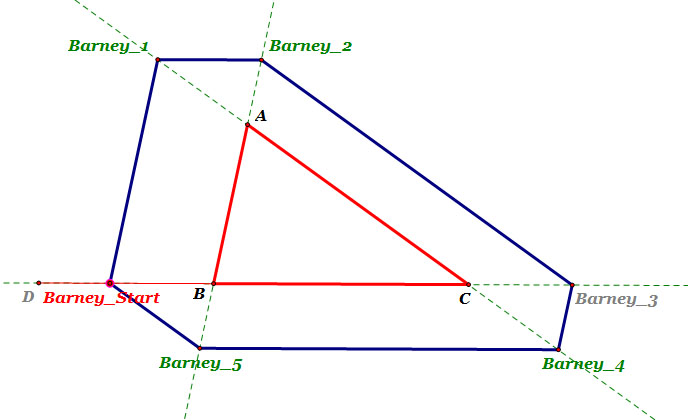
Case 5
: when Barney_Start is exterior to segment BC |
* If you want to see his trail (including exterior) in GSP movie, please click here.
>>> Barney's Trail Movie (including Exterior)
|
Conjecture
- The sum of length of Barney's trail is equal to the sum of the perimeter of triangle ABC and alpha*.
- Triangle A B_2 B_1, triangle B B_S B_5, and triangle C B_4 B_3 are similar to triangle ABC respectively, and all of them have same ratio of similitude.
* I will explain about the 'alpha' later.
|
|
Back to the Top |
Let's prove the conjecture from above. |
| Case 1 & 2
: when Barney_Start is interior to segment BC except at the midpoint |
|
|
| Conjecture |
The sum of length of Barney's trail is equal to the perimeter of triangle ABC. |
| Construction |
 |
| Proof |
Note the following:
- Since line segment AB is parallel to line segment B_1 B_S and line segment AC is parallel to line segment B_4 B_3,
quadrilateral A B_5 B_S B_1 is a parallelogram.
So,
m(line segment B_S B_1) = m(line segment A B_5).
- Since line segment BC is parallel to line segment B_2 B_1and line segment AB is parallel to line segment B_1 B_S,
quadrilateral B_2 B B_S B_1 is a parallelogram.
So,
m(line segment B_1 B_2) = m(line segment B B_S).
- Since line segment AC is parallel to line segment B_2 B_3 and line segment AB is parallel to line segment B_4 B_3,
quadrilateral A B_2 B_3 B_4 is a parallelogram.
So,
m(line segment B_2 B_3) = m(line segment A B_4).
- Since line segment BC is parallel to line segment B_5 B_4 and line segment AB is parallel to line segment B_4 B_3,
quadrilateral B_2 B B_S B_1 is a parallelogram.
So,
m(line segment B_3 B_4) = m(line segment B B_5).
- Since line segment BC is parallel to line segment B_5 B_4 and line segment AC is parallel to line segment B_5 B_S, quadrilateral C B_4 B_5 B_S is a parallelogram.
So,
m(line segment B_4 B_5) = m(line segment C B_S).
- Since line segment BC is parallel to line segment B_5 B_4 and line segment AC is parallel to line segment B_5 B_S,
quadrilateral C B_4 B_5 B_S is a parallelogram.
So,
m(line segment B_5 B_S) = m(line segment C B_4).
Since m(line segment A B_5) + m(line segment B_5 B) = m(line segment AB),
m(line segment B_S B_1) + m(line segment B_3 B_4) = m(line segment AB).
Since m(line segment C B_4) + m(line segment B_4 A) = m(line segment CA),
m(line segment B_5 B_S) + m(line segment B_2 B_3) = m(line segment CA).
Since m(line segment B B_S) + m(line segment B_S C) = m(line segment BC),
m(line segment B_1 B_2) + m(line segment B_4 B_5) = m(line segment BC).
Thus, total length of Barney's trail is
m(line segment B_S B_1) + m(line segment B_1 B_2) + m(mile segment B_2 B_3) + m(line segment B_3 B_4) + m(line segment B_4 B_5) + m(line segment B_5 B_S)
= [ m(line segment B_S B_1) + m(line segment B_3 B_4) ] + [ m(line segment B_5 B_S) + m(line segment B_2 B_3) ] + [ m(line segment B_1 B_2) + m(line segment B_4 B_5) ]
= m(line segment AB) + m(line segment BC) + m(line segment CA)
Hence, the sum of length of Barney's trail is equal to the perimeter of triangle ABC.
|
|
Case 3
: when Barney_Start is at the midpoint of segment BC |
|
| Conjecture |
The sum of length of Barney's trail is equal to half of the perimeter of triangle ABC. |
| Construction |
 |
| Proof |
Note the following:
- Since point B_S is the midpoint of line segment BC and point B_1 is the midpoint of line segment AB,
line segment B_S B_1 is the median of triangle ABC.
So, m(line segment B_S B_1) = 1/2 m(line segment CA).
- Since point B_1 is the midpoint of line segment AB and point B_2 is the midpoint of line segment CA,
line segment B_1 B_2 is the median of triangle ABC, too.
So, m(line segment B_1 B_2) = 1/2 m(line segmentBC).
- Since point B_2 is the midpoint of line segment CA and point B_S is the midpoint of line segment BC,
line segment B_2 B_S is the median of triangle ABC, too.
So, m(line segment B_2 B_S) = 1/2 m(line segment AB).
Thus, total length of Barney's trail is
m(line segment B_S B_1) + m(line segment B_1 B_2) + m(mile segment B_2 B_S)
= 1/2 m(line segment CA) + 1/2 m(line segment BC) + 1/2 m(line segment AB)
= 1/2 [ m(line segment CA) + m(line segment BC) + m(line segment AB) ]
Hence, the sum of length of Barney's trail is equal to half of the perimeter of triangle ABC.
* Note the following:
- m(line segment B_3 B_4) = m(line segment B_S B_1)
- m(line segment B_4 B_5) = m(line segment B_1 B_2)
- m(line segment B_5 B_S) = m(line segment B_2 B_S)
So, if we assume that the total length of Barney's trail is
m(line segment B_S B_1) + m(line segment B_1 B_2) + m(mile segment B_2 B_3) +m(line segment B_3 B_4) + m(line segment B_4 B_5) + m(line segment B_5 B_S),
the total length of Barney's trail would be equal to the perimeter of triangle ABC.
Therefore, we can conclude the following:
- When Barney_Start is interior to segment BC,
the total length of Barney's trail would be equal to the perimeter of triangle ABC.
|
|
Case 4
: when Barney_Start is on the end point of segment BC |
|
| Conjecture |
The sum of length of Barney's trail is equal to the perimeter of triangle ABC. |
| Construction |
 |
| Proof |
As we see, total length of Barney's trail is
m(line segment B_S B_1) + m(line segment B_1 B_2) + m(mile segment B_2 B_S)
= m(line segment CA) + m(line segment BC) + m(line segment AB)
Hence, the sum of length of Barney's trail is equal to the perimeter of triangle ABC.
* Note the following:
- m(line segment B_3 B_4) = m(line segment B_S B_1)
- m(line segment B_4 B_5) = m(line segment B_1 B_2)
- m(line segment B_5 B_S) = m(line segment B_2 B_S)
So, if we assume that the total length of Barney's trail is
m(line segment B_S B_1) + m(line segment B_1 B_2) + m(mile segment B_2 B_3) + m(line segment B_3 B_4) +m(line segment B_4 B_5) + m(line segment B_5 B_S),
the total length of Barney's trail would be equal to the perimeter of triangle ABC.
Therefore, we can conclude the following:
- When Barney_Start is on the end point of segment BC,
the total length of Barney's trail would be equal to two times of the perimeter of triangle ABC.
|
|
Case 5
: when Barney_Start is exterior to segment BC |
|
| Conjecture |
The sum of length of Barney's trail is equal to the sum of the perimeter of triangle ABC and alpha*.
Triangle A B_2 B_1, triangle B B_S B_5, and triangle C B_4 B_3 are similar to triangle ABC respectively, and all of them have same ratio of similitude. |
| Construction |
 |
| Proof |
1. Triangle A B_2 B_1, triangle B B_S B_5, and triangle C B_4 B_3 are similar to triangle ABC respectively.
Note the following:
- Since line segment BC is parallel to line segment B_1 B_2, triangle ABC and triangle A B_2 B_1 are similar.
So, m(line segment AB) : m(line segment A B_2)
= m(line segment BC) : m(line segment B_2 B_1)
= m(line segment CA) : m(line segment B_1 A)
- Since line segment CA is parallel to line segment B_S B_5, triangle ABC and triangle B_5 B B_S are similar.
So, m(line segment AB) : m(line segment B_5 B)
= m(line segment BC) : m(line segment B B_S)
= m(line segment CA) : m(line segment B_S B_5)
- Since line segment AB is parallel to line segment B_3 B_4, triangle ABC and triangle B_4 B_3 C are similar.
So, m(line segment AB) : m(line segment B_4 B_3)
= m(line segment BC) : m(line segment B_3 C)
= m(line segment CA) : m(line segment C B_4)
- Hence, triangle A B_2 B_1, triangle B_5 B B_S, and triangle B_4 B_3 C are also similar.
2. All of them have same ratio of similitude.
Note the following:
- Since line segment AB is parallel to line segment B_1 B_S and line segment B_1 B_2 is parallel to line segment B_5 B,
quadrilateral B_1 B_S B B_2 is a parallelogram.
So, m(line segment B_1 B_2) = m(line segment B_S B).
Thus, m(line segment BC) : m(line segment B_2 B_1) = m(line segment BC) : m(line segment B B_S)
Hence, triangle A B_2 B_1 and triangle B B_S B_5 are similar to triangle ABC, and they have same ratio of similitude.
- Since line segment CA is parallel to line segment B_2 B_3 and line segment B_1 B_2 is parallel to line segment C B_3,
quadrilateral B_1 C B_3 B_2 is a parallelogram.
So, m(line segment B_1 B_2) = m(line segment B_3 C).
Thus, m(line segment BC) : m(line segment B_2 B_1) = m(line segment BC) : m(line segment B_3 C)
Hence, triangle A B_2 B_1 and triangle B_4 B_3 C are similar to triangle ABC, and they have same ratio of similitude.
Therefore, triangle A B_2 B_1, triangle B B_S B_5, and triangle C B_4 B_3 are similar to triangle ABC respectively, and all of them have same ratio of similitude.
Hence, triangle A B_2 B_1, triangle B B_S B_5, and triangle C B_4 B_3 are congruent.
3. The sum of length of Barney's trail is equal to the sum of the perimeter of triangle ABC and alpha*.
The total length of Barney's trail is
m(line segment B_S B_1) + m(line segment B_1 B_2) + m(mile segment B_2 B_3) + m(line segment B_3 B_4) +m(line segment B_4 B_5) + m(line segment B_5 B_S)
= [ m(line segment AB) + m(line segment A B_2) ] + m(line segment B_1 B_2) + [ m(line segment B_1 A) + m(line segment CA) ] + m(line segment B_3 B_4) + [ m(line segment BC) + m(line segment B B_S) ] + m(line segment B_5 B_S)
Since triangle A B_2 B_1, triangle B B_S B_5, and triangle C B_4 B_3 are congruent,
m(line segment B_3 B_4) = m(line segment A B_2),
m(line segment B B_S) =m(line segment B_1 B_2),
m(line segment B_5 B_S) =m(line segment B_1 A).
So,
= [ m(line segment AB) + m(line segment A B_2) ] + m(line segment B_1 B_2) + [ m(line segment B_1 A) + m(line segment CA) ] + m(line segment A B_2) + [ m(line segment BC) + m(line segment B_1 B_2) ] + m(line segment B_1 A)
= [ m(line segment AB) + m(line segment BC + m(line segment CA) ] + [ 2 m(line segment A B_2) + 2 m(line segment B_1 B_2) + 2 m(line segment B_1 A) ]
= [ m(line segment AB) + m(line segment BC + m(line segment CA) ] + 2 [ m(line segment A B_2) + m(line segment B_1 B_2) + m(line segment B_1 A) ]
Thus, total length of Barney's trail is the sum of the perimeter of triangle ABC and two times of the perimeter of triangle A B_2 B_1;
however, remember that triangle ABC and triangle A B_2 B_1 are similar and they have a ratio of similitude.
So, the perimeter of triangle A B_2 B_1 = (ratio of the similitude) * (the perimeter of triangle ABC), and this is the alpha*.
Hence, total length of Barney's trail is the sum of the perimeter of triangle ABC and (ratio of the similitude) * (the perimeter of triangle ABC).
Therefore, we can conclude the following:
- When Barney_Start is exterior to segment BC,
the total length of Barney's trail would be equal to (the perimeter of triangle ABC) + (the ratio of the similitude) * (the perimeter of triangle ABC).
|
|
|
 Go Back to Top Go Back to Top |
 Go
Back to Michelle's Main page
Go
Back to Michelle's Main page |
 Go
Back to EMAT 6680 Homepage
Go
Back to EMAT 6680 Homepage |
Copyright
@ Michelle E. Chung |









